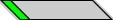Share
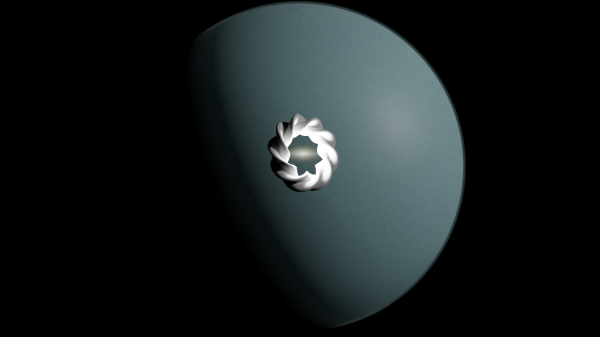Hawking's Knot
Hawkings' Knot
Image from Copyright Lilly Harper
This Hawking's Knot orbits Atlan in the Alpha Mensae system, where it converts low-value rubble into energy

Hi-tech Knots

Hawking's Knots are devices which use black holes to generate power from ordinary matter. The most basic type, a hi-tech process which can be built by advanced modosophont societies, consists of a small, artificially produced black hole of between ten million and a hundred million tonnes, which produces Hawking radiation at a predictable rate. For a ten million tonne black hole the luminosity is three terawatts, the lifetime before total evaporation is two million years, and the radius is 0.015 femtometres. These objects are almost always found in orbit for safety reasons, surrounded by a knot of energy collectors (hence the name).

Manufacturing such a small black hole is the most difficult part of the process, involving the forced collision of innumerable thin, massive rods at a speed very close to light speed, with a margin of error of about a nanometer. Attempts to create such objects were unsuccessful for many centuries, and even today can fail in a spectacular manner. For this reason artificial black holes are often obtained from transapientech sources, or may be manufactured by the sacrifice of magmatter (which itself can only be obtained as a gift from a transapient source). A specially shaped magmatter construct can be imploded into a black hole with relative ease. Once obtained, artificial black holes have many other uses, including gravity generators for artificial planets, and at the cores of Deep Well Industrial Zones.

Transapientech Knots

Seen from a distance, the workings of a transapientech Hawking's Knot can only be surmised; but current theory suggests that these devices consist of a very small, lightweight black hole nested in a complex geometry of small-scale space-time metric distorters, which are used to gather and reflect any emissions back into the hole, slowing the evaporation rate. The black hole's mass is controlled in such a fashion that it is near its evaporation point, and thus producing prodigious amounts of Hawking radiation. Effectively the hole is enclosed in an second, artificial event horizon which replicates that of a more massive hole.

When the Knot is "closed" or "tied," the distortion field works to redirect the flow of radiation back into the hole, thus maintaining its mass and preventing it from evaporating completely. When the Knot is "opened," the space-time metric is modulated to segregate antiparticles from particles and channel these away from the Knot to receiver/storage devices. Since this results in a decrease in the mass of the black hole, a constant stream of "feeder" particles is required to prevent the Knot from evaporating.

Well constructed Hawking Knots are fairly stable given a steady supply of fuel to counterbalance their loss of mass due to output and internal maintenance operations. They are operable by societies far below the level of technological sophistication required to produce them, and can provide a ready, cheap means of antimatter mass production.

One should be careful, though, not to "starve" the knot. Otherwise, the Knot may succumb to a runaway evaporation of its black hole. As radiation pressure overcomes the gravitational forces holding the distortion field geometry intact, the black hole loses mass at a higher and higher rate, radiating more and more intensely as it does, until it finally vanishes in a burst of gamma rays. Anyone interested in surviving such an event should be far away from the Knot when this happens.

As matter and antimatter are produced in roughly equal quantities through the evaporation process, the action of the system is to effectively convert constant streams of normal matter into equal amounts of matter and antimatter. Which particles are radiated depends on the temperature (T) of the black hole, which is related to its mass (M) by T = (h-bar) c^3/(8 pi G M k_b) where h-bar is Plank's reduced constant, c is the speed of light, G is the gravitational constant, and k_b is Boltzmann's constant. In
convenient units, this can be written as k_b T = 6.66E19 eV kg / M

The temperature expressed in energy units k_b T must be greater than the rest energy of the particle under consideration. Thus, for electron-positron creation, k_b T must be higher than 510,000 eV, and for proton-antiproton creation k_b T must be greater than 932,000,000 eV (more or less - there will be some pair production below this temperature, but it drops off rapidly). Therefore, a black hole cannot start producing protons and antiprotons until its mass gets as low as 70 million tons, while it can be producing electrons and positrons at 130 billion tons.

Hi-tech Hawking's Knots with a mass greater than 70 million tonnes will therefore only produce positrons, but smaller and (therefore hotter) examples will also produce anti-protons. Transapientech holes are hot enough to produce large quantities of anti-protons, as well as many other particles.

 
Related Articles
 
Appears in Topics
Matter ConversionMetric EngineeringPower Generation
Transapientech / Godtech / Clarketech
 
Development Notes
Text by David Jackson, with additions by Steve Bowers and Luke Campbell
Initially published on 19 April 2004.

 
Additional Information
Image 'Hawking's Knot' copyright by Lilly Harper used with permission. Please contact her for conditions of re-use at https://beaconsinthedark.wordpress.com/.
 
 
>